Question

A)

सीमा रेखाको समीकरण 2x + 3y = 6 र हल समूहमा
बिन्दु (0,0) भएको असमानता पत्ता लगाउनुहोस् ।
Find the inequality whose boundary line is
2x+3y= 6 and the solution set contains (0,0).​

Answer 1

Given:

The boundary line of an inequality is:

`2x+3y=6`

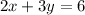
The solution set contains (0,0).

To find:

The inequality.

Solution:

The boundary line of an inequality is

`2x+3y=6`

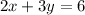
Since (0,0) is in the solution set, therefore (0,0) satisfies the required inequality.

Taking LHS, we get

`LHS=2x+3y`

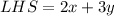
Substitute x=0 and y=0.

`LHS=2(0)+3(0)`

`LHS=0+0`

`LHS=0`

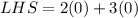
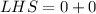
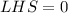
The right hand side of the given equation is 6.

`RHS=6`

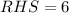
We know that,

`0<6`

`LHS<RHS`

`2x+3y<6`

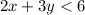
The boundary line is not a dotted line, it means the points on the boundary line are also included in the solution set. So, the inequality sign must be
`\leq`.

`2x+3y\leq 6`

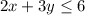
Therefore, the required inequality is
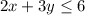`2x+3y\leq 6`.