Question

Pls helppp anyoneeee plssss

Answer 1

Given:

Mean = 45

Standard deviation = 9

Confidence level = 95%.

To find:

The confidence interval.

Solution:

The formula for confidence interval is:

`C.I.=\overline{x}\pm z(\sigma)/(√(n))`

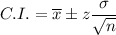
Where,
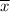`\overline{x}` is mean, z is the z-value at given level of confidence,
`\sigma` is the standard deviation and n is the number of observations.

The z-value at 95% confidence level is 1.96.

Here number of observations are not given. Assume it is 1.

Putting
`\overline{x}=45,\sigma=9,z=1.96,n=1`.

`C.I.=45\pm 1.96(9)/(√(1))`

`C.I.=45\pm 1.96(9)`

`C.I.=45\pm 17.64`

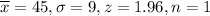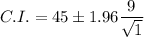
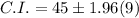
It can be written as

`C.I.=[45-17.64,45+17.64]`

`C.I.=[27.36,62.64]`

Approximate the value to the nearest whole number.

`C.I.=[27,63]`

The interval for the middle 95% of snowfall is 27 to 63.

Therefore, the correct option is A.