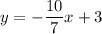Question

The points (7, –7) and (0, 3) fall on a particular line. What is its equation in slope-intercept form?

Answer 1

`\displaystyle y=-(10)/(7)x+3`

Explanation:

We want to find the equation of the line that includes the points (7, -7) and (0, 3).

First, we will find the slope of the line using the slope formula given by:

`\displaystyle m=(y_2-y_1)/(x_2-x_1)`

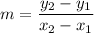
So, the slope of our line is:

`\displaystyle m=(3-(-7))/(0-7)=(10)/(-7)=-(10)/(7)`

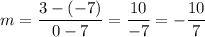
Next, notice that the given point (0, 3) is our y-intercept.

Slope-intercept form is given by:

`y=mx+b`

Where m is the slope and b is the y-intercept.

Therefore, by substitution, we acquire that our equation is:

`\displaystyle y=-(10)/(7)x+3`