Question

You invested ​$18,000 in two accounts paying 7% and 8% annual​ interest, respectively. If the total interest earned for the year was $1290 how much was invested at each​ rate?

Answer 1

$15,000 was invested at 7%

$3,000 was invested at 8%

Explanation:

Let
`x` be the amount of money you spend on the 7% account and
`y` be the amount of money you spend on the 8% account. With the information given, you can set up two equations:

`x+y=18000`

`0.07x+0.08y=1290`

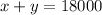
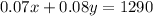
Rearrange the first equation and multiply the second equation to get:

`x=18000-y`

`7x+8y=129000`

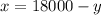
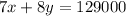
Then, substitute
`x` for
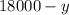`18000-y` in the second equation to get:

`7(18000-y)+8y=129000`

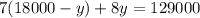
Use the distributive property (
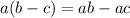`a(b-c)=ab-ac`) to get:

`126000-7y+8y=129000`

Subtract
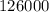`126000` from both sides and combine like terms to get:

`y=3000`

Thus, substitute
`y` for
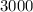`3000` in the first original equation to get:

`x+3000=18000`

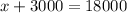
Finally, subtract
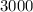`3000` from both sides to reach:

`x=15000`

Our answer is $15,000 was invested at 7% and $3,000 was invested at 8%.

Hope this helps :)

Answer 2

• 8%: $3000
• 7%: $15000

Explanation:

Let x represent the amount invested at 8%. Then the amount invested at 7% is (18000-x) and the interest earned is ...

0.08x +0.07(18000 -x) = 1290

0.01x +1260 = 1290 . . . simplify

0.01x = 30 . . . . . . . . . . subtract 1260

x = 3000 . . . . . . . . . . multiply by 100

$3000 was invested at 8%; $15000 was invested at 7%.