Given:
Central angle of a sector = 72 degrees
Intercepted arc length = 44 cm
To find:
The radius of the circle.
Solution:
We know that, the arc length is
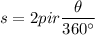
Where, r is the radius of the circle,
 is the central angle in degrees.
is the central angle in degrees.
Putting
 and
and
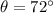 , we get
, we get
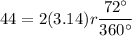
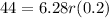
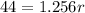
Divide both sides by 1.256.

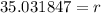
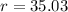
Therefore, the radius of the circle is about 35.03 cm.