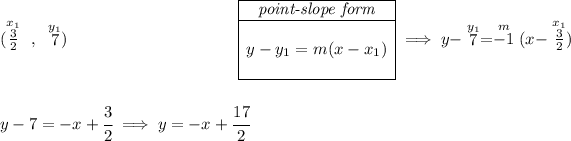neverminding the jumbled lingo, is simply asking for the equation of the tangent line at that point, it says all tangents, well, there's only one passing there.
we can simply get the derivative of f(x) and take it from there.

since now we know the slope when x = 3/2, then we can just plug that into its point-slope intercept form, along with the coordinates.