Question

A software engineer creates a LAN game where an 8 digit code made up of 1,2,3,4,5,6,7,8 has to be decided on, universal code . There is a condition that each number has to be used a and no number can be repeated . What is the probability that first 4 digits of the code are even numbers ?

Answer 1

0.0143 = 1.43% probability that first 4 digits of the code are even numbers.

Explanation:

Arrangements of n elements:

The number of possible arrangements of n elements is given by:

`A_(n) = n!`

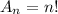
Probability:

A probability is the number of desired outcomes divided by the number of total outcomes.

In this question:

Desired outcomes:

First four digits are even(2, 4, 6 and 8 are even, so arrangements of 4 elements).

Last four digits are odd(1, 3, 5 and 7 are odd, so the last four elements are also arrangements of 4 elements). So

`D = 4!*4! = 24*24 = 576`

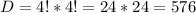
Total outcomes:

Arrangements of 8 digits. So

`T = A_(8) = 8! = 40320`

Probability:

`p = (D)/(T) = (576)/(40320) = 0.0143`

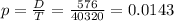
0.0143 = 1.43% probability that first 4 digits of the code are even numbers.