Question

PLS ANSWER ITS URGENT

Suppose a new regulation states that the maximum angle of a ramp for wheelchairs is 9º. At least how

long must the new ramp be? Round to the nearest tenth of a foot.

Answer 1

The length of the ramp must be at least 14.71 feet

Explanation:

Given

`BC = 2.3ft`

`\theta = 9^\circ`

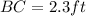
See attachment for complete figure

Required

Length of the ramp (z)

To do this, we consider sin of angle A (
`\theta`).

So, we have:

`sin\theta = (Opposite)/(Hypotenuse)`

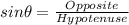
This gives:

`sin(9)= (2.3)/(z)`

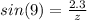
Make z the subject

`z= (2.3)/(sin(9))`

`z = (2.3)/(0.1564)`

`z = 14.71 ft`

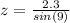
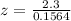
The length of the ramp must be at least 14.71 feet