Answer:
L = 116.6 m
Step-by-step explanation:
For this exercise we approximate the tunnel as a tube with one end open and the other closed, at the open end there is a belly and at the closed end a node, therefore the resonances occur at
λ = 4L 1st harmonic
λ = 4L / 3 third harmonic
λ = 4L / 5 fifth harmonic
General term
λ = 4L / n n = 1, 3, 5,... odd
n = (2n + 1) n are all integers
They indicate that two consecutive resonant frequencies were found, the speed of the wave is related to the wavelength and its frequency
v = λ f
λ = v / f
we substitute
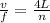
L =
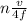
for the first resonance n = n
L = (2n + 1)
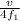
for the second resonance n = n + 1
L = (2n + 3)
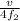
we have two equations with two unknowns, let's solve by equating
(2n + 1) \frac{v}{4f_1}= (2n + 3) \frac{v}{4f_2}
(2n + 1) f₂ = (2n +3) f₁
2n + 1 = (2n + 3)
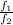
2n (1 - \frac{f_1}{f_2}) = 3 \frac{f_1}{f_2} -1
we substitute the values
2n (1-
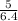 ) = 3
) = 3
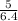 -1
-1
2n 0.21875 = 1.34375
n = 1.34375 / 2 0.21875
n = 3
remember that n must be an integer.
We use one of the equations to find the length of the Tunal
L = (2n + 1) \frac{v}{4f_1}
L = (2 3 + 1)
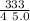
L = 116.55 m