Answer:
0.36 = 36% probability that the land has oil and the test predicts it
Explanation:
Conditional Probability
We use the conditional probability formula to solve this question. It is

In which
P(B|A) is the probability of event B happening, given that A happened.
 is the probability of both A and B happening.
is the probability of both A and B happening.
P(A) is the probability of A happening.
45% chance that the land has oil.
This means that
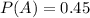
He buys a kit that claims to have an 80% accuracy rate of indicating oil in the soil.
This means that
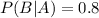
What is the probability that the land has oil and the test predicts it?
This is
 . So
. So

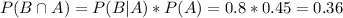
0.36 = 36% probability that the land has oil and the test predicts it