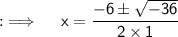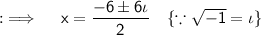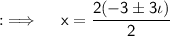Question

Which of the following is a solution of x^2+6x=-18

Answer 1

We are provided with a quadratic equation , and we have to find it's roots , but let's recall some things

• The Standard Form of a quadratic equation is ax² + bx + c = 0

• If D > 0 , then the equation have two real and distinct roots/zeroes .

• If D = 0 , then the equation have two equal roots/zeroes .

• If D < 0 , then the equation have two imaginary roots/zeroes or you can say that no real roots

Where , D is the Discriminant whose value is b²- 4ac and the roots of the equation are given by a very famous formula called The Quadratic Formula , which is as follows ;

`{\quad {\boxed{\pmb{\bf x = (-b \pm √(D))/(2a)}}}}`

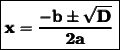
Now , coming back on the question ;

We are provided with the equation x² + 6x = -18 , so on comparing this equation with the standard form of quadratic equation , we have , a = 1 , b = 6 and c = 18 . Now calculating the Discriminant ;

`{: \implies \quad \sf D=(6)^(2)-4* 1 * 18}`

`{: \implies \quad \sf D=36-72}`

`{: \implies \quad \sf D=-18}`

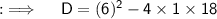
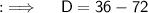
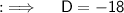
As , D < 0 , so two imaginary roots exist . Now by quadratic formula ;

`{: \implies \quad \sf x=(-6 \pm √(-36))/(2* 1)}`

`{: \implies \quad \sf x=\frac{-6 \pm \sqrt{\underline{6* 6}* -1}}{2}}`

`{: \implies \quad \sf x=(-6 \pm 6√(-1))/(2)}`

`{: \implies \quad \sf x=(-6 \pm 6\iota)/(2) \quad \{\because √(-1)=\iota\}}`

`{: \implies \quad \sf x=(2(-3\pm 3\iota))/(2)}`

`{\quad \qquad \boxed{\bf \therefore \: x=-3 \pm 3\iota}}`