Question

Functions f(x) and g(x) are shown:

f(x) = x2 g(x) = x2 + 8x + 16

In which direction and by how many units should f(x) be shifted to match g(x)?
A. Left by 4 units
B. Right by 4 units
C. Left by 8 units
D. Right by 8 units

Answer 1

Explanation:

Factor g(x)

(x+4)(x+4)

(x+4)^2

So f(x) needs to be shifted left by 4 units.

Answer 2

Option A, Left by 4 units

Explanation:

Step 1: Convert g(x) to a function square

We currently have g(x) in this order:
`ax^2 + bx + c`

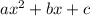
However, we want g(x) to be in this order:
`(ax + c)^(2)`

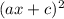
The first thing we have to do is to factor it out:

`g(x)=x^(2)+8x+16`

`g(x) = (x + 4)(x + 4)`

`g(x) = (x+4)^(2)`

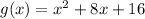
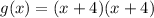
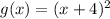
Step 2: Now we can see which way we need to move it

The original form is:
`f(x) = (ax - b)^(2)`

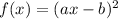
Since the - has changed to a +, that means that we moved -4 spaces down the x-axis. This means that we move left by 4 units.

Answer: Option A, Left by 4 units

Look at the graphs below to make sure: