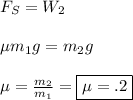Hi there!
Assuming the pulley is frictionless and massless, we can do a summation of forces for each block.
Block 1 has the tension force (in direction of acceleration) and force of static friction acting on it. For the block to be stationary, the net force must be 0, so:
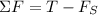
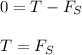
Now, we can do a summation for Block 2.
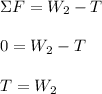
We can substitute this tension in the above equation to solve for the force of static friction and the coefficient of static friction.