Question

The surface area of this cube is 864 square yards. What is the value of q?

Answer 1

Volume

=

512

cubic feet

Explanation:

A cube has

6

faces all with equal area.

If the total surface area of the cube is

384

sq.ft.

then each face has an area of

384

÷

6

=

64

sq.ft.

Each face of a cube is a square and if we denote the length of an edge as

E

then the area of a face (in this case

64

sq.ft.) is equal to

E

2

XXX

E

2

=

64

sq.ft.

XXX

→

E

=

8

sq.ft.

The volume of a cube with edges of length

E

is

E

3

In this case

Volume

=

E

3

=

8

3

=

512

sq.ft.

Hope i helped

Answer 2

The value of q is 12 yards.

To find the value of q, let's use the formula for the surface area of a cube:

`\[ \text{Surface Area} = 6s^2 \]`

where s is the length of a side of the cube.

Given that the surface area is 864 square yards, we can set up an equation:

`\[ 6s^2 = 864 \]`

Now, solve for s:

`\[ s^2 = (864)/(6) \]`

`\[ s^2 = 144 \]`

`\[ s = 12`

Since q represents the length of a side of the cube,
`\( q = s = 12 \)`.

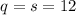Therefore, the value of q is 12 yards.