Question

Find f such that f'(x) = 8x – 3. f(4) = 0​

Answer 1

Answer: y=29 / (4,29)

Explanation:

By graphing
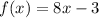`f(x)=8x-3` and
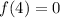`f(4)=0` on Desmos. You'll be able to find that when x is 4, y is 29.

Similarly, you can plugin 4 into the original equation (
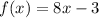`f(x)=8x-3`) Which looks like:

`8(4)-3\\32-3=29`

Additionally, you can change
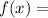`f(x)=` to
`y=` as it is the exact same thing. With that in mind, you can do the same with
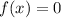`f(x)=0` and just change it to x=4. As you're wanting to know what the y-value is when x=4.

Answer 2

4x²-3x+c is our original equation

Explanation:

we have an independent number I called this number c

put 4 from x and try to find c

f(4)=4*(4²)-3*(4)+c=0

we have to be careful about f(4) is 0

64-12+c=0 and c is -52 so our original equation is 4x²-3x-52