Answer:
1) The angle of projection of the ball is approximately 52.496° above the horizontal
2) The velocity with which the boy throws the ball to enable him accomplish his desire is approximately 14.769 m/s
Step-by-step explanation:
In the projectile motion of the ball, the parameters are;
The distance the boy stands from the front of the house = 5 m
The depth of the house, front to back = 6 m
The height of the opening of the front window above him = 5 m
The height of the back window = 2 m higher than the front window
Therefore, the height of the back window = 5 m + 2 m = 7 m
1) The general formula for the vertical height of a parabolic projectile motion is given as follows;
y = a·x² + b·x + c
At y = 0, x = 0, therefore, c = 0
At y = 5, x = 5, we have;
5 = a·5² + b·5
∴ 5 = 25·a + 5·b...(1)
At y = 7, x = 11, we have;
7 = a·11² + b·11
7 = 121·a + 11·b...(2)
Making 'a' the subject of equation (1) and (2) and equating both values of 'a' gives;
For equation (1);
a = (5 - 5·b)/25 = 1/5 - b/5
a = 1/5 - b/5
For equation (2);
a = (7 - 11·b)/121 = 7/121 - b/11
a = 7/121 - b/11
∴ 1/5 - b/5 = 7/121 - b/11
1/5 - 7/121 = b/5 - b/11
86/605 = 6·b/55
b = (86/605) × (55/6) = 43/33
b = 43/33
a = 1/5 - b/5 = 1/5 - (43/33)/5 = -2/33
∴ y = (-2/33)·x² + (43/33)·x
The slope of the curve = dy/dx = d((-2/33)·x² + (43/33)·x)/dx = -4/33·x + 43/33
The slope of the curve at any point on the projectile = -4/33·x + 43/33
The slope at the origin is given by plugging x = 0 as follows
The slope at the origin = -4/33 × 0 + 43/33 = 43/33
The slope at the origin = tan(θ) = 43/33
Where;
θ = The angle of projection of the ball
∴ θ = arctan(43/33) ≈ 52.496°
The angle of projection of the ball, θ ≈ 52.496° above the horizontal
2) At the back widow, the equation for the vertical height, 'h', is given as follows;

Where;
h = The vertical height of the back window = 7 m
tan∠θ = 43/33
x = The horizontal distance of the back window from the boy = 5 m + 6 m = 11 m
g = The acceleration due to gravity = 9.8 m/s²
v₁ = The velocity with which the boy throws the ball
cos²∠θ = cos²(arctan(43/33)) = 1089/2938
Plugging in the values gives;
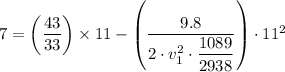

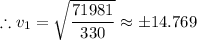
The velocity with which the boy throws the ball to enable him accomplish his desire, v₁ ≈ 14.769 m/s.