Question

Two particles, an electron and a proton, are initially at rest in a uniform electric field of magnitude 570 N/C. If the particles are free to move, what are their speeds (in m/s) after 47.6 ns?

Answer 1

Step-by-step explanation:

Given that,

Two particles, an electron and a proton, are initially at rest in a uniform electric field of magnitude 570 N/C.

We need to find their speeds after 47.6 ns.

For electron,

The electric force is given by :

`F=qE\\\\F=1.6* 10^(-19)* 570\\\\=9.12* 10^(-17)\ N`

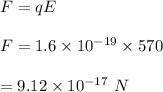
Let a be the acceleration of the electron. So,

F = ma

m is mass of electron

`a=(F)/(m)\\\\a=(9.12* 10^(-17))/(9.1* 10^(-31))\\\\a=10^(14)\ m/s^2`

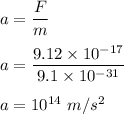
Let v be the final velocity of the electron. So,

v = u +at

u = 0 (at rest)

So,

`v=10^(14)* 47.6* 10^(-9)\\\\v=4.76* 10^6\ m/s`

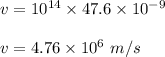
For proton,

Acceleration,

`a=(9.12* 10^(-17))/(1.67* 10^(-27))\\\\=5.46* 10^(10)\ m/s^2`

Now final velocity of the proton is given by :

`v=5.46* 10^(10)* 47.6* 10^(-9)\\\\v=2598.96\ m/s`

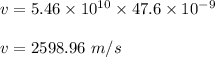
Hence, this is the required solution.