Question

A ball is thrown vertically downward from the top of a 37.4-m-tall building. The ball passes the top of a window that is 15.4 m above the ground 2.00 s after being thrown. What is the speed of the ball as it passes the top of the window?

Answer 1

v= 20.8 m/s

Step-by-step explanation:

• Assuming no other forces acting on the ball, from the instant that is thrown vertically downward, it's only accelerated by gravity, in this same direction, with a constant value of -9.8 m/s2 (assuming the ground level as the zero reference level and the upward direction as positive).
• In order to find the final speed 2.00 s after being thrown, we can apply the definition of acceleration, rearranging terms, as follows:

`v_(f) = v_(o) + a*t = v_(o) + g*t (1)`

• We have the value of t, but since the ball was thrown, this means that it had an initial non-zero velocity v₀.
• Due to we know the value of the vertical displacement also, we can use the following kinematic equation in order to find the initial velocity v₀:

`\Delta y = v_(o) *t + (1)/(2) * a* t^(2) (2)`

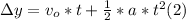
• where Δy = yf - y₀ = 15.4 m - 37.4 m = -22 m (3)

• Replacing by the values of Δy, a and t, we can solve for v₀ as follows:

`v_(o) = ((\Delta y- (1)/(2) *a*t^(2)))/(t) = (-22m+19.6m)/(2.00s) = -1.2 m/s (4)`

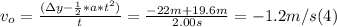
• Replacing (4) , and the values of g and t in (1) we can find the value that we are looking for, vf:

`v_(f) = v_(o) + g*t = -1.2 m/s - (9.8m/s2*2.00s) = -20.8 m/s (5)`

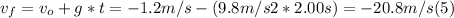
• Therefore, the speed of the ball (the magnitude of the velocity) as it passes the top of the window is 20.8 m/s.