Question

4 points

Untitled Question
Kimberly is asked to find mZM. What is her error?
N
sin M _ sin
16 10
sin M=
16.sin 33"
10
16
10
m2M = 0.8714
X Х
33
M
What is Kimberly's error?
A Kimberly did not find the inverse cosine of the value she calculated.
OB. Kimberly did not find the inverse sine of the value she calculated.
OC. Kimberly found the measure of angle N, not M.
D. Kimberly made a computational error.
E Kimberly did not apply the Law of Sines correctly
А

Answer 1

The error is;

B. Kimberly did not find the inverse sine of the value she calculated

Explanation:

The given parameters of the triangle ΔLMN are;

`\overline {LN}` = 16

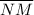`\overline {NM}` = 10

∠L = 33°

By sine rule, we have;

`(sin \ M)/(16) = (sin \ L)/(10) = \frac{sin \ N}{\overline {LM}}`

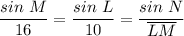
Given that ∠L = 33°, we have;

`(sin \ M)/(16) = (sin \ 33^(\circ))/(10)`

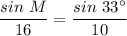
Therefore, we have;

`sin \ M = 16 * (sin \ 33^(\circ))/(10) \approx 0.8714`

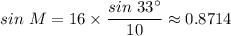
sin M ≈ 0.8714

`\therefore m \angle M = sin^(-1) \, (sin \ M)\approx sin^(-1) (0.8714) \approx 60.62^(\circ)`

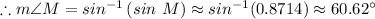
Therefore, m∠M ≈ 60.62°

m∠M ≠ sin M ≈ 0.8714

Therefore;

Kimberly did not find the inverse sine (sin⁻¹) of the value she calculated