1 Answer

Jayesh Lathiya · Answer 1 · 2022-09-28T01:38:02+0000

Given:

Point A'(6,3) is a dilation of point A(18,9).

To find:

The scale factor.

Solution:

We know that, if a point P is dilated by factor k and origin is the center of dilation, then

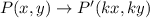
$P(x,y)\to P'(kx,ky)$

Let the scale factor for the given problem be k. So,

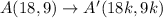
$A(18,9)\to A'(18k,9k)$

On comparing A'(18k,9k) and A'(6,3), we get

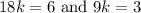
$18k=6\text{ and }9k=3$

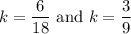
$k=(6)/(18)\text{ and }k=(3)/(9)$

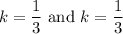
$k=(1)/(3)\text{ and }k=(1)/(3)$

Therefore, the scale factor is
.

Please log in or register to add a comment.