Answer:
As given,
I =
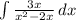
Since the degree of the numerator is less than the degree of the denominator, we don't need to divide.
We factor the denominator as x(x-2)
The partial fraction decomposition is
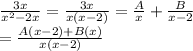
we get
3x = A(x-2) + B(x)
⇒3x = x(A+B) - 2A
By comparing , we get
A+ B = 3 , -2A = 0
⇒A = 0 and B = 3- A = 3-0 = 3
∴ we get
A = 0, B = 3
Therefore, the integral become
![\int\limits {(3x)/(x^(2) - 2x) } \, dx = \int\limits {[(0)/(x) + (3)/(x-2) ] } \, dx](https://img.qammunity.org/2022/formulas/mathematics/college/k7ukoq0lfbaea9l6xnfrrr3be8cswbv1ji.png)