Answer:
Because
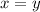 , then the solution is unique.
, then the solution is unique.
Explanation:
Given
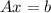

See comment for complete question
Let there be two solutions x and y.
Such that:
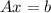 and
and
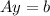
Since:
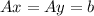
Then:

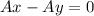
This implies that:
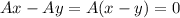
The only trivial solution is:
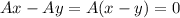
Such that:

Then
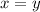
Because
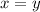 , then the solution is unique.
, then the solution is unique.