Given:
In quadrilateral EFGH,
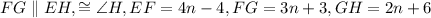
To find:
The length of segment GH.
Solution:
Draw a figure according to the given information as shown below.
In quadrilateral EFGH,
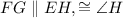 , it means the quadrilateral EFGH is an isosceles quadrilateral because base angles are equal.
, it means the quadrilateral EFGH is an isosceles quadrilateral because base angles are equal.
Now, quadrilateral EFGH is an isosceles quadrilateral, so the sides EF and GH are equal.
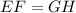
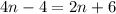
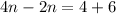
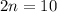
Divide both sides by 2.
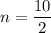
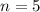
Now,
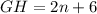
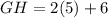
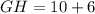
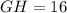
Therefore, the correct option is C.