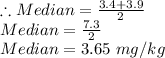Question

Arsenic in Toenails Arsenic is toxic to humans, and people can be exposed to it through contaminated drinking water, food, dust, and soil. Scientists have devised an interesting new way to measure a person’s level of arsenic poisoning: by examining toenail clippings. In a recent study,1 scientists measured the level of arsenic (in mg/kg) in toenail clippings of eight people who lived near a former arsenic mine in Great Britain. The following levels were recorded: 0.8 1.9 2.7 3.4 3.9 7.1 11.9 26.0 1Button, M., Jenkin, G.,Harrington, C., and Watts, M., ‘‘Human Toenails as a biomarker of exposure to elevated environment arsenic,’’ Journal of Environmental Monitoring, 2009; 11(3): 610–617. Data are reproduced from summary statistics and are approximate.

(a) Do you expect the mean or the median of these toenail arsenic levels to be larger?
Mean
Median
(b) Calculate the mean. Round your answer to one decimal place. Meanequals mg/kg Calculate the median.
Enter the exact answer. Median equals mg/kg

Answer 1

The answers are:

a) I expect the mean to be larger

b) Mean = 7.2 mg/kg

Median = 3.65 mg/kg

Explanation:

Writing out the distribution for clarity:

0.8 1.9 2.7 3.4 3.9 7.1 11.9 26.0

a) I expect the mean to be larger because from the distribution, the median (middle number) will be less than 4 ( 0.8 1.9 2.7 | 3.4 3.9 | 7.1 11.9 26.0) and the mean will be greater because, from the distribution of the numbers, it is expected to be greater than 4, which the median does not attain.

b) Mean: is a measure of central tendency, that is the average of all the numbers in a distribution. It is calculated by adding up all the number in the distribution and dividing by the number of terms, as follows:

`Mean (\bar x) = (0.8 + 1.9 + 2.7 + 3.4 + 3.9 + 7.1 +11.9 + 26.0)/(8) \\\bar x = (57.7)/(8) \\\bar x = 7.2\ mg/kg`

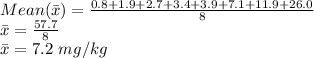
∴Mean = 7.2 ( to one decimal place)

Median: This is the middle term in a distribution. When the number of distribution is odd, the middle term is picked, or when even, the average of the two middle terms is taken after arranging the numbers in ascending or descending order. This is shown as follows:

0.8 1.9 2.7 3.4 3.9 7.1 11.9 26.0 (two middle term boldened)

`\therefore Median = (3.4 +3.9)/(2) \\Median = (7.3)/(2)\\Median = 3.65\ mg/kg`