Question

Write the equation of the line that passes through the points (2, 3) and (3, -7). Put

your answer in fully reduced point-slope form, unless it is a vertical or horizontal line.

Answer 1

`y - 3 = -10 (x-2)`

Explanation:

The point-slope formula is
`y-y_1 = m (x-x_1)`. When knowing the slope of a line and a point it intersects, we can write its equation with that formula. Substitute
`m`,
`x_1`, and
`y_1` for real values.

1) First, find
`m`, or the slope. Use the slope formula
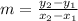`m = (y_2-y_1)/(x_2-x_1)` and substitute the x and y values of the given points. Then, solve:

`m = ((-7)-(3))/((3)-(2)) \\m = (-7-3)/(3-2) \\m = (-10)/(1) \\m = -10`

Therefore, the slope of the line is -10.

2) Now, substitute the needed real values into the point-slope formula,
`y-y_1 = m (x-x_1)`. Since
`m` represents the slope, substitute -10 in its place. Since
`x_1` and
`y_1` represent the x and y values of a point the line crosses, choose any of the points given and substitute its values into the formula. (Either one is fine, they both equal the same thing. I chose (2,3).) This will give the following answer and equation:

`y - 3 = -10 (x-2)`