Final Answer:
The probability of getting all heads or all tails in five tosses of the loaded coin is
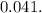
Step-by-step explanation:
For a loaded coin with a probability of landing heads in a single toss as \(5/6\) and \(1/6\) for tails, we're determining the probability of obtaining either all heads or all tails in five tosses.
The probability of getting all heads in five tosses is \((5/6)^5\) because the events are independent. Similarly, the chance of getting all tails in five tosses is \((1/6)^5\). The probability of getting all heads or all tails is the sum of these two individual probabilities.

Understanding probability in simple events like coin tossing demonstrates the application of probability rules, especially with independent events. Such exercises help to comprehend the chances of specific outcomes occurring over multiple trials.