The general solution to the given differential equation is
 .
.
Substitution:
Define
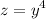 .
.
Substitute z into the equation, resulting in

Factoring the Quadratic:
Factor the quadratic as
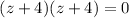
Therefore,
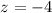
Back-Substitution:
Substitute z back into
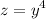 , yielding
, yielding
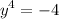
Finding Roots:
Take the fourth root of both sides, but consider complex roots:
![$y= \pm \sqrt[4]{-4}=\pm \sqrt[4]{4} \cdot \sqrt[4]{-1} y= \pm 2 \cdot( \pm i)= \pm 2 i, \pm 2 i$](https://img.qammunity.org/2024/formulas/mathematics/college/ko8iwvewng7uelrb64qv0g53i7m509lits.png)
Therefore, the general solution to the differential equation is
 .
.
Key Point:
- The original equation involves
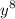 , indicating the possibility of complex roots.
, indicating the possibility of complex roots. - Taking the fourth root of a negative number introduces imaginary units (
 ).
). - The general solution involves complex numbers, not real numbers.