Question

Which quadratic expression represents the product of these factors? (2x+5)(7-4x)

Answer 1

The quadratic expression that represents the product of the factors (2x+5)(7-4x) is -8x² - 6x + 35.

Step-by-step explanation:

The quadratic expression that represents the product of the factors (2x+5)(7-4x) is:

(2x)(7) + (2x)(-4x) + (5)(7) + (5)(-4x)

Simplifying, we have:

14x - 8x² + 35 - 20x

Combining like terms, we get:

-8x² - 6x + 35

Answer 2

The quadratic expression that represents the product of the factors (2x + 5)(7 - 4x) is
`-8x^2 - 6x + 35`.

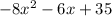How to find the quadratic expression

To find the quadratic expression that represents the product of the factors (2x + 5)(7 - 4x), use the distributive property of multiplication.

`(2x + 5)(7 - 4x)` can be expanded as follows:

`(2x)(7) + (2x)(-4x) + (5)(7) + (5)(-4x)`

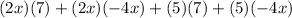
Simplifying each term:

`14x - 8x^2 + 35 - 20x`

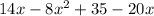
Rearranging the terms in descending order of powers:

`-8x^2 + (14x - 20x) + 35`

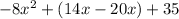
Combining like terms:

`-8x^2 - 6x + 35`

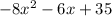
Therefore, the quadratic expression that represents the product of the factors (2x + 5)(7 - 4x) is
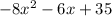`-8x^2 - 6x + 35`.