Question

Solve for ∠R, to the nearest degree

Answer 1

`\angle R \approx 58^(\circ)`

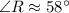
Explanation:

The Law of Cosines states that for any triangle ABC, where A, B, and C are angles and a, b, and c are sides:
`c^2 = a^2 + b^2 -2ab\cos C`.

Since we wish to find R, we can use the law of cosines:

`8^2 = 7^2 + 9^2 - 2\cdot7\cdot9\cos R`

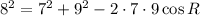
Solving for cos R, we find it to be 11/21. Taking the inverse cosine of this value, we find angle R to have a measure of 58.411864° ≈ 58°.

Answer 2

∠R= 58.4°

Explanation:

For this question, we have to use cosine law. Cosine law is used when there is 3 known side and one unknown angle and there is 1 known angle, two known sides and one unknown side. Memorize the cosine law for unknown angle formula for the unknown angle:

x=
`Cos^(-1)`(
`(a^(2)+b^(2)-c^(2) )/(2ab)`) (I'll attach it also)

Here x is the unknown angle and in the attachment it is gamma. a and b can be any of the adjacent sides to x. c is the opposite side of x.

Now plug in the values:

x= Cos^{-1}((9²+7²-8²)/2(9×7))

x= 58.41186°= 58.4°