Question

Question 3 3.75 pts What is the value today of receiving $5,000 at the end of each six-month period for the next four years, assuming an annual interest rate of 4% compounded semi-annually? O $34,512. O $36,627. O $32,459. O $33,664. O $40,000 3.75 pts

Answer 1

The value today of receiving $5,000 at the end of each six-month period for the next four years, assuming an annual interest rate of 4% compounded semi-annually, is $33,664.

Step-by-step explanation:

To calculate the value of a series of cash flows with compound interest, we use the formula:

`V = P[(1 + r/n)^(nt) - 1] / (r/n)`

In this case, P is the present value (which is zero since we are finding the future value), r is the annual interest rate (4%), n is the number of times interest is compounded per year (2), and t is the number of years (4).

First, we calculate the future value of each $5,000 payment:

`FV = P*(1 + r/n)^(nt)`

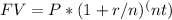
FV = 5,000*(1 + 0.02)^(2*4)

FV = $32,459 per payment

Next, we add up all the future values to get the total value:

V = FV1 + FV2 + FV3 + FV4

V = $32,459 * 4 = $129,836

However, we need to discount this amount back to today's value using the same formula:

`V = P[(1 + r/n)^(nt) - 1] / (r/n)V = 0[(1 + 0.02/2)^(2*4) - 1] / (0.02/2)`

V = $33,664.09 (rounded to two decimal places)

Therefore, the total value today of receiving $5,000 at the end of each six-month period for the next four years with an annual interest rate of 4% compounded semi-annually is $33,664.