Question

HELP ASAP!!!

3 Questions.
Identify whether the function graphed has an odd or even degree and a positive or negative leading coefficient.

Answer 1

If a graph directs in the same direction(upward or downward), it's of an even degree, and vice versa. If it increases, it has a positive sign in the leading term, and the opposite is true.

3 of 6: even; negative

5 of 6: odd; negative

6 of 6: odd; positive

Principle

But you might want to see how it works, don't you? Let's see it, the limits!

`\boxed{x > 1\implies 1 < x < {x}^(2) < {x}^(3) < \cdots}`

`\boxed{x < -1\implies\cdots < -x^(3) < {x}^(2) < -x < 1}`

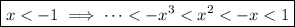
If the function heads toward the right infinity, it keeps the same direction. Okay, this is easy to understand, but what about another one? The sign alternates. The odd degree makes a turn!

What if the coefficient is significantly larger than the previous one?

Let's consider the coefficient. Since
`|x|\to\infty`, no matter how greater the coefficient, its absolute value will be less than
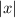`|x|`, which keeps the priority of the increment behind, so nothing matters!