Answer:
20 units
Explanation:
L = Length of rectangle = Side VW
= Side YX
=
 units
units
W = Width of rectangle = Side WX
= Side VY
=
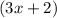 units
units
Perimeter of rectangle = 88 units
Perimeter of rectangle = Sum of exterior sides
=
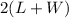 units
units
![88 = 2[(4x) + (3x + 2)]](https://img.qammunity.org/2024/formulas/mathematics/high-school/niypx2t6i9bwtx6600ochmv3whlt3dm92l.png)
![88 = 2[4x + 3x + 2]](https://img.qammunity.org/2024/formulas/mathematics/high-school/3kqwbsgc6hij3xwg5rh0ax21m092al3fo2.png)
![88 = 2[7x + 2]](https://img.qammunity.org/2024/formulas/mathematics/high-school/rw2j7185iibkr4s3s968pmtpj1i7xht3ef.png)
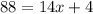
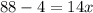
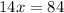
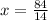
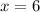
Substituting the calculated value of ‘x’ in the expression for the width of the rectangle:
Length of Side WX =
![[3(6) + 2]](https://img.qammunity.org/2024/formulas/mathematics/high-school/o3ktgdhtbtsan9gj230rkumuy5bk5x4hpi.png) units
units
=
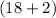 units
units
= 20 units