Question

!50 POINTS! (3 SIMPLE GEOMETRY QUESTIONS)

QUESTIONS BELOW
|
|
\/

Answer 1

Note qn 2 and 3 are the same so answer for both is the same

I hope this helps pls ask if in doubt.

Answer 2

x = 12.1

x = 3

Explanation:

From observation of the given right triangles, we can see that their interior angles are 30°, 60° and 90°. Therefore, they are special 30-60-90 triangles.

The side lengths in a 30-60-90 triangle have a special relationship which can be represented by the ratio 1 : √3 : 2.

The formula for the side lengths is a : a√3 : 2a, where "a" represents a scaling factor that can be any positive real number.

• Side a is opposite the 30° angle (shortest leg).
• Side a√3 is opposite the 60° angle (longest leg).
• Side 2a is the hypotenuse (longest side).

This ratio holds true regardless of the scale of the triangle. Therefore, if we know the length of one side of a 30-60-90 triangle, we can calculate the length of the other sides using this ratio.

`\hrulefill`

In triangle one, the hypotenuse is c = 14. As 2a is the hypotenuse, we can use this to calculate the scale factor "a".

`2a = 14 \implies a = 7`

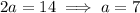
The side labelled "x" is opposite the 60° angle, and is therefore a√3. Therefore:

`\begin{aligned}x&=a√(3)\\&=7√(3)\\&=12.1\; \sf (nearest\;tenth)\end{aligned}`

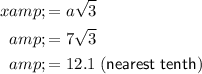
Therefore, the value of x is 12.1, rounded to the nearest tenth.

`\hrulefill`

In triangle two, the hypotenuse is c = 6. As 2a is the hypotenuse, we can use this to calculate the scale factor "a".

`2a =6 \implies a = 3`

The side labelled "x" is opposite the 30° angle, and is therefore simply "a". Therefore:

`x=a=3`

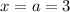
Therefore, the value of x is 3.

Note: Triangles 2 and 3 are the same.