Question

Please help it's due tomorrow ​

Answer 1

B. -414,720 x⁷y⁶

Explanation:

To find the 4th term of the expansion of (2x - 3y²)¹⁰, we can use the binomial theorem.

The binomial theorem states that for an expression of the form (a + b)ⁿ:

`\displaystyle (a+b)^n=\binom{n}{0}a^(n-0)b^0+\binom{n}{1}a^(n-1)b^1+...+\binom{n}{r}a^(n-r)b^r+...+\binom{n}{n}a^(n-n)b^n\\\\\\\textsf{where }\displaystyle \rm \binom{n}{r} \: = \:^(n)C_(r) = (n!)/(r!(n-r)!)`

For the expression (2x - 3y²)¹⁰:

• a = 2x
• b = -3y²
• n = 10

Therefore, each term in the expression can be calculated using:

`\displaystyle \boxed{\binom{n}{r}(2x)^(10-r)(-3y^2)^r}\quad \textsf{where $r = 0$ is the first term.}`

The 4th term is when r = 3. Therefore:

`\begin{aligned}\displaystyle &\;\;\;\;\:\binom{10}{3}(2x)^(10-3)(-3y^2)^3\\\\&=(10!)/(3!(10-3)!)(2x)^7(-3y^2)^3\\\\&=(10!)/(3!\:7!)\cdot2^7x^7(-3)^3y^6\\\\&=120\cdot 128x^7 \cdot (-27)y^6\\\\&=-414720\:x^7y^6\\\\ \end{aligned}`

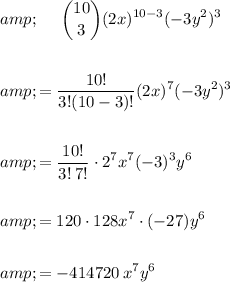
So the 4th term of the given expansion is:

`\boxed{-414720\:x^7y^6}`