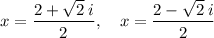Question

Solve the equation 2x^2-4x+3=0 using the quadratic formula.

Answer 1

The quadratic formula is -b + or - sqrt(b^2-4ac) /2a

For this equation a=2 b=-4 c=3

When you replace the values you get
4+ or - sqrt(-4^2 -4(2)(3) ) /2(2)

First you should simplify what is being square rooted
-4^2 -4(2)(3) = 16 - 24 = -8

now you have 4+ or - sqrt(-8) / 2x2 or 4

Now you can divide the 4 by the 4 which just cancels out and leaves 1

So you should now have 1+ or - sqrt(-8) and since -8 cannot be square rooted you know that the parabola does not touch the x-axis meaning there are no REAL solutions to this equation or no zeros in other words.

Answer: x=1+- sqrt(-8)

Hope this made sense! this is a hard one to explain.

Answer 2

`x=(2+ √(2)\:i)/(2), \quad x=(2-√(2)\:i)/(2)`

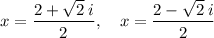
Explanation:

Quadratic formula

The quadratic formula is a mathematical formula used to solve quadratic equations. It provides the solutions for an equation of the form ax² + bx + c = 0, where a, b, and c are constants, and x represents the variable.

The quadratic formula is given by:

`\boxed{x=(-b \pm √(b^2-4ac))/(2a)\quad\textsf{where }\:ax^2+bx+c=0}`

`\hrulefill`

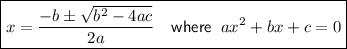
Given quadratic equation:

`2x^2 - 4x+3=0`

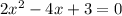
To solve the given quadratic equation using the quadratic formula, first identify the coefficients a, b, and c in the given equation:

• a = 2
• b = -4
• c = 3

Substitute the values of a, b, and c into the quadratic formula and solve for x:

`x=(-(-4) \pm √((-4)^2-4(3)(2)))/(2(2))`

`x=(4 \pm √(16-24))/(4)`

`x=(4 \pm √(-8))/(4)`

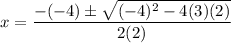
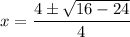
Since the expression under the square root is negative, we have complex solutions:

`x=(4 \pm √(8\cdot(-1)))/(4)`

`x=(4 \pm √(8)√(-1))/(4)`

`x=(4 \pm 2√(2)\:i)/(4)`

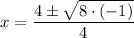
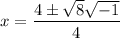
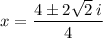
Simplify by dividing the numerator and denominator by 2:

`x=(2\pm √(2)\:i)/(2)`

`\hrulefill`

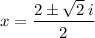
Solutions

Therefore, the solutions to the equation 2x² - 4x + 3 = 0 are:

`x=(2+ √(2)\:i)/(2), \quad x=(2-√(2)\:i)/(2)`