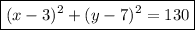Question

NO LINKS! URGENT HELP PLEASE!

Write the equation of the following circles​

Answer 1

`\textsf{a)} \quad (x+1)^2+(y-4)^2=36`

`\textsf{b)} \quad (x-5)^2+(y+2)^2=64`

`\textsf{c)} \quad (x-3)^2+(y-7)^2=130`

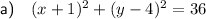
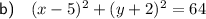
Explanation:

To write the equation of a circle given its center and radius, we can substitute the values into the standard circle equation formula.

`\boxed{\begin{minipage}{5 cm}\underline{Equation of a circle}\\\\$(x-a)^2+(y-b)^2=r^2$\\\\where:\\ \phantom{ww}$\bullet$ $(a, b)$ is the center. \\ \phantom{ww}$\bullet$ $r$ is the radius.\\\end{minipage}}`

Part a

Given values:

• Center (a, b) = (-1, 4)
• Radius r = 6

Substitute the values into the circle equation formula:

`(x-(-1))^2+(y-4)^2=6^2`

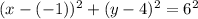
Therefore, the equation of the circle is:

`\boxed{(x+1)^2+(y-4)^2=36}`

`\hrulefill`

Part b

Given values:

• Center (a, b) = (5, -2)
• Diameter = 16

The diameter of a circle is twice its radius.

Therefore, if the diameter of the circle is 16, the radius is r = 8.

Substitute the values into the circle equation formula:

`(x-5)^2+(y-(-2))^2=8^2`

Therefore, the equation of the circle is:

`\boxed{(x-5)^2+(y+2)^2=64}`

`\hrulefill`

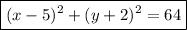
Part c

Given values:

• Center (a, b) = (3, 7)
• Point on the circle = (-4, -2)

Substitute the values into the circle equation formula and solve for r²:

`(-4-3)^2+(-2-7)^2=r^2`

`(-7)^2+(-9)^2=r^2`

`49+81=r^2`

`r^2=130`

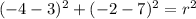
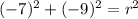
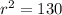
Therefore, the equation of the circle is:

`\boxed{(x-3)^2+(y-7)^2=130}`