Question

Simplify (4^-2)^5 I have no clue what the answer is

Answer 1

1/ 4^10

(ONE on top of a fraction. Four to the tenth power on the bottom of the fraction)

Explanation:

When you have a power to a power the short cut (exponent rule) says to multiply the powers.

(4^-2)^5

Keep the same base, the 4

times -2 × 5, you get -10

so far after one step, you have

4^-10

Now usually, you would not leave an exponent negative. So to "fix" the negative exponent, you change it to positive by "pushing it" across the fraction bar.

4^-10

becomes:

1/ 4^10

This is a ONE on top of a fraction and FOUR to the tenth power on the bottom of the fraction.

Answer 2

The answer is:

1/4¹⁰

Work/explanation:

For this problem, I'm going to use the following exponent law:

`\boxed{\!\!\boxed{\quad\sf{(x^m)^n=x^(mn)}\quad}\!\!}`

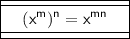
So if we have "a power to a power", we multiply the powers.

And now that we're familiar with this property let's apply it.

`\sf{(4^(-2))^5=4^(-2*5)=4^(-10)}`

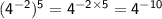
Here the next exponent law comes into play.

______________________

`\boxed{\!\!\boxed{\quad\sf{x^(-m)\:\:=\:\:(1)/(x^m)\quad}}\!\!}`

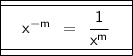
So if we have a number raised to a negative power, we flop it over.

Let's apply the law to our problem now.

Our problem is:

`\sf{4^(-10)`

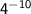
According to the law above, we should do the following:

`\sf{4^(-10)=(1)/(4^(10))}`

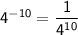
It's better to leave the answer as it is.

Hence, the answer is 1/4¹⁰