Answer:

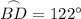
Explanation:
The given diagram shows a circle with diameter BC and radius OD (where O is the center of the circle).
Angles on a straight line sum to 180°. Therefore:
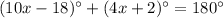
Solving the equation for x:
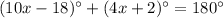
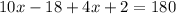
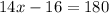
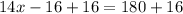
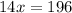

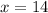
Therefore, the value of x is 14.
The measure of an arc is equal to the measure of its corresponding central angle.
To find the measure of arc BD, substitute the found value of x into the expression of the arc's corresponding central angle.
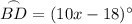

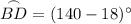
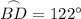
Therefore, the measure of arc BD is 122°.