Question

The time taken by Nick to do his statistics homework follows a normal distribution with a mean of 25 minutes and a standard deviation of 5 minutes. Jasmin’s homework time also follows a normal distribution with a mean of 50 minutes and a standard deviation of 10 minutes. They hate each other’s guts and never work together so we can assume that Nick’s homework completion is independent of Jasmine’s homework completion.

a) Define the random variable D to be the difference between the amount of time spent on a randomly selected assignment of Jasmin’s and a randomly selected assignment of Nick’s. So D = N – J. Find the mean and the standard deviation of D.

b) Calculate the probability that Nick spent longer doing his assignment than Jasmin did. Show your method clearly.

Answer 1

a) Mean of D = -25

Standard deviation of D = 11.18

b) P(N > J ) = 0.9875

Explanation:

Given - μₙ = 25, σₙ = 5 , μₐ = 50 , σₐ = 10

where μₙ is mean of nick and μₐ is mean of jasmine

σₙ = 5 is standard deviation of nick and σₐ is standard deviation of jasmine

To find - a) Define the random variable D to be the difference between the amount of time spent on a randomly selected assignment of Jasmine’s and a randomly selected assignment of Nick’s. So D = N – J. Find the mean and the standard deviation of D.

b) Calculate the probability that Nick spent longer doing his assignment than Jasmine did. Show your method clearly.

Proof -

a)

Mean of D = Mean of nick - Mean of jasmine

= 25 - 50 = -25

standard deviation of D = √Standard deviation of Nick + jasmine

= √5² + 10² = √25 + 100 = √125 = 11.18

∴ we get

Mean of D = -25

Standard deviation of D = 11.18

b)

We have to find - P(N > J)

If N > J , then D > 0

Now,

The z-score is expressed as

z =
`(0 - (-25))/(11.18) = (25)/(11.18) = 2.24`

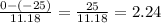
Now,

P(N>J) = P (D > 0) = P(Z < 2.24)

= 0.9875

⇒P(N > J ) = 0.9875