Question

An FCC iron-carbon alloy initially containing 0.20 wt% C is carburized at an elevated temperature and in an atmosphere wherein the surface carbon concentration is maintained at 1.0 wt%. If after 48 h the concentration of carbon is 0.35 wt% at a position 3.9 mm below the surface, determine the temperature at which the treatment was carried out. You will need to use data in the two tables below to solve this problem.

Answer 1

Hello the table required is missing attached below is the missing table

Answer : 1299.05 k

Step-by-step explanation:

Given data :

concentration of carbon ( Cx ) at a position 3.9mm below surface = 0.35

concentration of carbon ( Co ) = 0.20

surface carbon ( Cs ) = 1

Determine the temperature at which the treatment was carried out

first we will determine the value of Z in the table attached

given that the value of erf ( Z ) for Z = 0.8125 from the table

=
`(Z- 0.9)/(0.95-0.9) = (0.8125-0.797)/(0.8209-0.797)`

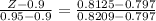
make Z subject of the equation

Z = 0.932

next calculate the diffusion coefficient using the relation below

Z =
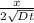`(x)/(2√(Dt) )` ----- ( 1 ) where ; z = 0.932 , x = 3.9 mm , t = 48h

0.932 =

x = 3.9 mm = 0.0039 m

t = 48 h = ( 48 *60* 60 ) = 172800 secs

Insert values into equation 2

0.932 =
`(0.0039)/(2√(D * 172800) )`

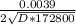
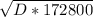`√(D*172800)` = (0.0039 / 0.932 ) / 2

172800 * D =( 0.0021 )^2

therefore D = 2.55 * 10^-11 m^2/s

Finally calculate the temperature at which the treatment was carried out

D =
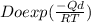`Do exp((-Qd)/(RT) )` ----- ( 3 )

D = 2.55 * 10^-11 m^2/s ,

Do = 2.3*10^-5 m^2/s ( gotten from Diffusion data table )

Qd = 148000 J/mol ( gotten from Diffusion data table )

R = 8.31 J/mol k ( gotten from Diffusion data table )

back to equation 3

D / Do = exp ( -17810 / T )

1.11 * 10^-6 = exp ( -17810 / T )

therefore T = -17810 / ln( 1.11 * 10^-6 )

= - 17810 / -13.71 = 1299.05 k