Question

Stephan has a box in the shape of a hexagonal prism where the hexagonal bases are regular. A net of the box is below.

In the hexagon diagram, all the sides are drawn with a rectangle on every side and each is added and another hexagon is added to a rectangle.

Note: Figure is not drawn to scale.

He measured the height of the box to be 7 in. Then, Stephan drew a line from the center of one of the hexagons to each of its vertices and noticed that all the triangles he created have a height of 9 in and a base of 10 in.

The geometry of the hexagon is equally divided into six parts of the triangles are shaded inside. The top side is marked as 10 inches, and the length of the triangle is 9 inches.

Note: Figure is not drawn to scale.

What is the surface area of the hexagonal prism?
A.
750 sq in
B.
690 sq in
C.
960 sq in
D.
1,380 sq in

Answer 1

To find the surface area of the hexagonal prism, we need to find the area of the two hexagonal bases and the area of the six rectangular sides.

The area of one of the hexagonal bases is:

area = (3√3 / 2)(10 in)^2
area = 259.8 sq in

The area of the other hexagonal base is the same, so the total area of the bases is 2(259.8 sq in) = 519.6 sq in.

The area of each of the rectangular sides is:

area = height x base
area = 7 in x 10 in
area = 70 sq in

There are six rectangular sides, so the total area of the rectangular sides is 6(70 sq in) = 420 sq in.

Therefore, the total surface area of the hexagonal prism is:

surface area = 519.6 sq in + 420 sq in
surface area = 939.6

The closest answer choice is C. 960 sq in, so that would be the best choice.

Answer 2

C. 960 sq in.

To find the surface area of the hexagonal prism, we need to find the areas of all the surfaces and then add them up.

Hexagonal Bases:

Each hexagonal base has an area given by the formula:

`A_(hexagon)` =
`(3√(3) )/(2) x side^(2)` =
`(3√(3) )/(2) x 10^(2)`

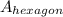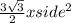Lateral Faces (Rectangles):

There are six rectangular lateral faces. Each rectangle's area is given by

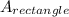`A_(rectangle)` =length×width = 6×(10×7).

Triangles on the Sides:

There are six identical triangles on the sides. Each triangle's area is given by
`A_(triangle)` =
`(1)/(2)` ×base×height = 6 x
`(1)/(2)` x 10 x 9

Now, let's calculate these areas and add these areas to get the total surface area

Total Surface Area ≈960sq in