Question

Suppose a normal distribution has a mean of 222 and a standard deviation of

16. What is the probability that a data value is between 206 and 230? Round
your answer to the nearest tenth of a percent.
A. 91.0%
B. 66.9%
C. 53.3%
D. 84.0%

Answer 1

C) 53.3%

The probability that a data value is between 206 and 230

P( 206 ≤X≤230) = 0.5328 = 53.3%

Explanation:

Explanation

Given that Mean of the Normal distribution(μ) = 222

Given that the standard deviation of the Normal distribution (σ) = 16

Let 'X' be the random variable in the Normal distribution

we have to find that the probability that a data value is between 206 and 230

solution:-

Step(i):-

Let 'X' = 206

`Z = (x^(-)-mean )/(S.D) = (206-222)/(16) = -1`

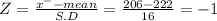
Let X = 230

`Z = (x-mean )/(S.D) = (230-222)/(16) = 0.5`

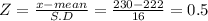
Step(ii):-

The probability that a data value is between 206 and 230

P( 206 ≤X≤230) = P( -1≤Z≤0.5)

= |A(0.5)+A(-1)|

= 0.1915+0.3413

= 0.5328

final answer:-

The probability that a data value is between 206 and 230

P( 206 ≤X≤230) = 0.5328 = 53.3%