Answer:
2035.752 in³/min
Explanation:
To find the rate of change of the volume of the sphere at the instant its radius is 9 inches, we need to work out dV/dt when r = 9.
The equation for the volume of a sphere is:
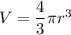
Differentiate the expression for volume with respect to r:
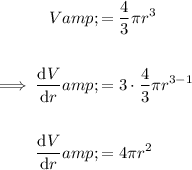
We know that that the radius of a sphere is increasing at a constant rate of 2 inches per minute, so the rate of change of the radius of sphere is:
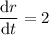
To find an expression for dV/dt, use the chain rule:

Substitute the expressions for dV/dr and dr/dt to create an expression for dV/dt:

To find the value of dV/dt when r = 9, substitute r = 9 into the equation for dV/dt:
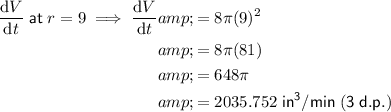
Therefore, the rate of change of the volume of the sphere at the instant when its radius is 9 inches is 2035.752 in³/min (rounded to three decimal places).