Answer:
The true statements about the function and its graph are:
- The value of f(-10) = 82.
- The graph of the function is a parabola.
- The graph contains the point (20, -8).
Step-by-step explanation:
Given quadratic function:
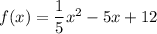
When a quadratic function is graphed on the coordinate plane, the function forms a curve called a parabola.
If the leading co-efficient of the quadratic function is positive, the parabola opens upwards. If the leading co-efficient is negative, the parabola opens downwards.
As the leading co-efficient of the given quadratic function is positive, the parabola opens upwards.
To find the value of f(-10), substitute x = -10 into the function:
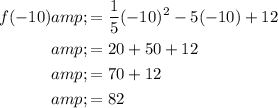
Therefore, f(-10) = 82.
To determine if the graph contains the point (20, -8), substitute x = 20 into the function:
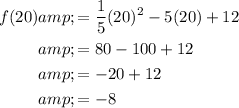
Therefore, the graph contains the point (20, -8).
To determine if the graph contains the point (0, 0), substitute x = 0 into the function:

Therefore, the graph contains the point (0, 12), not point (0, 0).
The true statements about the function and its graph are:
- The value of f(-10) = 82.
- The graph of the function is a parabola.
- The graph contains the point (20, -8).