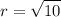Question

Find the radius of the circle. The center of the circle is (2, -3) and a point that lies on the circle is (-1, -2).

Answer 1

`\displaystyle r = √(10)`

General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Algebra I

• Coordinates (x, y)

Geometry

• Definition of a radius - the center of a circle to any point to the circumference

Algebra II

• Distance Formula:
  `\displaystyle d = √((x_2-x_1)^2+(y_2-y_1)^2)`

Explanation:

Step 1: Define

Center (2, -3) → x₁ = 2, y₁ = -3

Circumference point (-1, -2) → x₂ = -1, y₂ = -2

In this case, the distance d from the center to the circumference point would be the radius r of the circle.

Step 2: Find Radius r

1. [Distance Formula] Define equation [Radius]:
   `\displaystyle r = √((x_2-x_1)^2+(y_2-y_1)^2)`
2. Substitute in points [Radius]:
   `\displaystyle r = √((-1-2)^2+(-2--3)^2)`
3. [Radius] [√Radical] (Parenthesis) Simplify:
   `\displaystyle r = √((-1-2)^2+(-2+3)^2)`
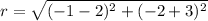
4. [Radius] [√Radical] (Parenthesis) Subtract/Add:
   `\displaystyle r = √((-3)^2+(1)^2)`
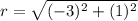
5. [Radius] [√Radical] Evaluate exponents:
   `\displaystyle r = √(9+1)`
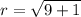
6. [Radius] [√Radical] Add:
   `\displaystyle r = √(10)`