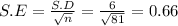Answer:
a) t-statistic t = 0.9532
b) The standard error S.E = 1.2
c) The new t-statistic = 1.95
d) The new estimated standard error =0.666
Explanation:
Step(i):-
Given that the mean of the Population = 16
The sample size n=27
The mean of the sample = 17.1
Given that the variance of the sample (S²) = 36.0
The standard deviation of the sample (S) = √36 = 6
Test statistic
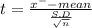
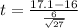
t = 0.9532
b)
The standard error is defined by
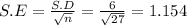
Step(ii):-
c) given that the sample size n = 81
Test statistic
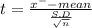
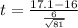
t = 1.65
d)
The new standard error is defined by