Final answer:
The distance between the points P(4,-3) and Q(-3,5) is approximately sqrt(113) units.
Step-by-step explanation:
To find the distance between two points in a Cartesian plane, we can use the distance formula. The formula is:
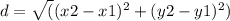
Using the given points, P(4,-3) and Q(-3,5), we can substitute the values into the formula:
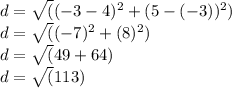
So, the distance between the points P(4,-3) and Q(-3,5) is approximately
 units.
units.