Answer:
The period of the model is
 seconds. The period represents the time needed for the function to complete one cycle.
seconds. The period represents the time needed for the function to complete one cycle.
Explanation:
Cosine is a trigonometric function and trigonometric functions are characterized by having a periodical behavior. The period is the time needed for the function to cover an angle of
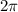 radians. By this approach we find that:
radians. By this approach we find that:
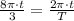 (1)
(1)
Where:
 - Time, measured in seconds.
- Time, measured in seconds.
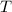 - Period, measured in seconds.
- Period, measured in seconds.
Then, we solve (1) for
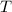 :
:
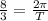

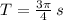
The period of the model is
 seconds. The period represents the time needed for the function to complete one cycle.
seconds. The period represents the time needed for the function to complete one cycle.