Question

Imagine you are a financial analyst at an investment bank. According to your research of publicly-traded companies, 60% of the companies that increased their share price by more than 5% in the last three years replaced their CEOs during the period. At the same time, only 35% of the companies that did not increase their share price by more than 5% in the same period replaced their CEOs. Knowing that the probability that the stock prices grow by more than 5% is 4%, find the probability that the shares of a company that fires its CEO will increase by more than 5%.

Answer 1

0.0667 = 6.67% probability that the shares of a company that fires its CEO will increase by more than 5%.

Explanation:

Conditional Probability

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

In which

P(B|A) is the probability of event B happening, given that A happened.

`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

In this problem:

Event A: Company fires the CEO

Event B: Shares increase by more than 5%.

Probability of a company firing it's CEO:

35% of 100 - 4 = 96%(shares did not increase by more than 5%).

60% of 4%(shared did increase by more than 5%).

So

`P(A) = 0.35*0.96 + 0.6*0.04 = 0.36`

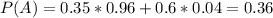
Intersection of events A and B:

Fires the CEO and shared increased by more than 5%, is 60% of 4%. So

`P(A \cap B) = 0.6*0.04 = 0.024`

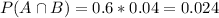
Probability that the shares of a company that fires its CEO will increase by more than 5%.

`P(B|A) = (P(A \cap B))/(P(A)) = (0.024)/(0.36) = 0.0667`

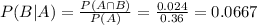
0.0667 = 6.67% probability that the shares of a company that fires its CEO will increase by more than 5%.