Final Answer:
1. Pendulum Bob's Equation: The equation for the pendulum bob's horizontal location is
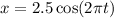 , where
, where
 represents the distance from the equilibrium position in centimeters, and
represents the distance from the equilibrium position in centimeters, and
 is the time in seconds.
is the time in seconds.
2. Bob's Position after 3.2 seconds: After 3.2 seconds, the bob is located approximately 1.85 centimeters to the right of the equilibrium position.
Step-by-step explanation:
For a pendulum bob swinging in small arcs with a width of 5 centimeters and a period of 1 second, the equation describing its horizontal location
 is given by
is given by
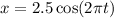 . Here, the amplitude
. Here, the amplitude
 is half the width of the arc, so
is half the width of the arc, so
 centimeters. The cosine function oscillates between -1 and 1, with the maximum displacement being the amplitude
centimeters. The cosine function oscillates between -1 and 1, with the maximum displacement being the amplitude
 .
.
To find the bob's position after 3.2 seconds, substitute
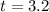 into the equation:
into the equation:
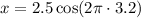
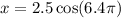
 (since cosine of
(since cosine of
 is 1)
is 1)
 centimeters
centimeters
Therefore, after 3.2 seconds, the bob is 2.5 centimeters to the right of the equilibrium position.
This result aligns with the periodic nature of the cosine function, where at
 , the bob starts at the farthest right position (maximum positive displacement), and every 1-second interval corresponds to a complete cycle of the cosine function. The position oscillates between the positive and negative values of the amplitude
, the bob starts at the farthest right position (maximum positive displacement), and every 1-second interval corresponds to a complete cycle of the cosine function. The position oscillates between the positive and negative values of the amplitude
 , which is 2.5 centimeters in this case.
, which is 2.5 centimeters in this case.